

EQUATIONS CONTAINING VARIABLES UNDER ONE OR MORE RADICALS

Note::
Example 5: ![]()
Since the index of the radical is even, the quantity under the
radical cannot be negative and 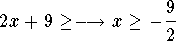 .
.
Subtract 18 from both sides of the equation so that the radical
term is isolated.
![]()
At this point you observe that the positive left side of the
equation cannot equal the negative number on the right side of the
equation, you are through. There is no solution. If you did not make
this observation, let's continue.
Divide both sides by 3 and then square both sides of the equation.
![]()
(2x+9)=4
2x=-5
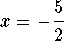
The answer is 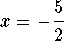
Check the solution by substituting ![]() in the original equation
in the original equation
for x. If the left side of the equation equals the right side of the
equation after the substitution, you have found the correct answer.
Left side:
![]()
![]()
3*2+18
6+18
=24
Right side: Since the left side of the equation does not equal the right side of
the original equation when you substitute your answer, your answer is
not the solution. There is no solution.
You can also check the answer by graphing
![]()
(the left side of the original equation minus the right side of the
original equation). The solution will be the x-intercept. There is no x-
intercept, and hence there is no solution.
If you would like to test yourself by working some problems similar to
this example, click on problem Links to S2211
If you would like to go back to the equation table of contents, click

 S.O.S. MATHematics home
page
S.O.S. MATHematics home
pageDo you need more help? Please post your question on our S.O.S. Mathematics CyberBoard.

Author: Nancy Marcus