


The ``forget the minus sign" definition of the absolute value is useless for our purposes. Instead, we will mostly use the geometric definition of the absolute value:
The absolute value of a number measures its distance to the origin on the real number line.
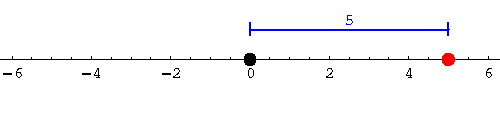
Since 5 is at 5 units distance from the origin 0, the absolute value of 5 is 5, |5|=5
|
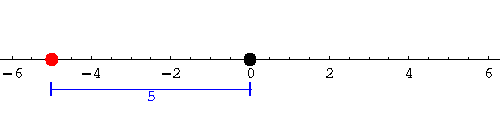
Since -5 is also at a distance of 5 units from the origin, the absolute value of -5 is 5, |-5|=5:
|
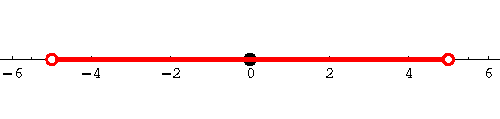
We are ready for our first inequality. Find the set of solutions for
Translate into English: we are looking for those real numbers x whose distance from the origin is less than 5 units.
Obviously we are talking about the interval (-5,5):
|
What about the solutions to ![]() ?
?
In English: which numbers, x, are at least 2 units away from the origin? On the left side, real numbers less than or equal to -2 qualify, on the right all real numbers greater than or equal to 2:

|
We can write this interval notation as
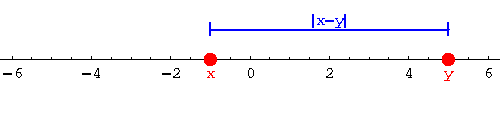
What is the geometric meaning of |x-y|?
|x-y| is the distance between x and y on the real number line.
|
Consider the example |(-4)-3|. The distance on the real number line
between the points -4 and +3 is 7, thus
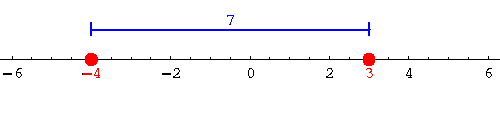
|
Let's find the solutions to the inequality:

|
We're talking about the numbers in the interval [1,3].
What about the example
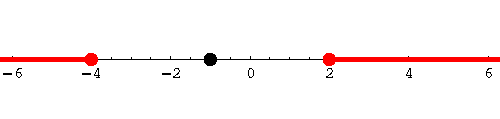
|
The set of solutions is
With a little bit of tweaking, our method can also handle inequalities
such as

Thus we obtain
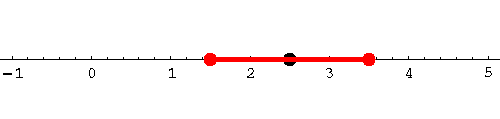
|
So the original inequality has as its set of solutions the interval
![]() .
.
Consider the example
Our method fails for more contrived examples.
Let us consider the inequality
The standard definition for the absolute value function is given by:
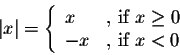
We will do exactly that!
Let's first consider only those values of x for which ![]() :
:
Case 1: ![]()
In this case we know that |x-3|=x-3, so our inequality becomes
x is a solution if ![]() and x>1 at the same time! We're talking
about numbers
and x>1 at the same time! We're talking
about numbers ![]() .
.
What if x-3<0?
Case 2: x<3
This time x-3<0, so
|x-3|=-(x-3)=3-x,
so our inequality reads as
Under our case assumption x<3, solutions are those real numbers
which satisfy
![]() .
.
We're talking about numbers in the interval

 S.O.S MATHematics home page
S.O.S MATHematics home page Do you need more help? Please post your question on our S.O.S. Mathematics CyberBoard.

Helmut Knaust